The half-life determines how quickly a radioisotope decays
Map of nuclear half-lives
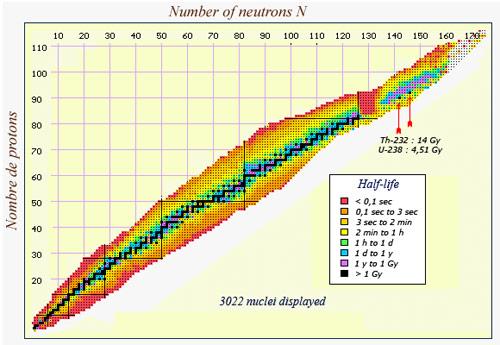
This map of nuclei shows the extraordinary range of half-lives that exists in nature. Along the central line drawn in black, one finds the nuclei that are either stable or have a half-live longer than a billion years. Nuclei which survive for a fraction of a second, however, (coloured in red) can be found on the borders of the map. A group of very heavy, very unstable nuclei can also be found around the 130 neutron, 85 proton mark. Among the heavier nuclei, one finds an island of relative stability (in mauve), where a group of isotopes have a half-life between one and a billion years. The two heaviest natural such nuclei, thorium 232 and uranium 238, are the solitary black dots in the mauve island.
© NUCLEUS
The ‘half-life’ of a radioactive nucleus is one of its main features with the nature of radiations it emits. It determines how quickly it will decay and for how long we need to worry about its radiations. Half-lives can range from a fraction of a second to billions of years.
Three natural nuclei have half-lives above one billion years (Potassium 40, uranium-238 and thorium-232) while the half-life of polonium 214, a descendant of the same uranium-238, is only 0,16 millisecond.
The longer the half-life of a nucleus, the lower the radioactive activity. A nucleus with a half-life that is a million times greater than another will be a million times less radioactive.
A ‘half-life’ is defined as the amount of time taken for the number of nuclei present in a sample at a given time to exactly halve. This value does not depend on the moment chosen: the amount of time taken for the nuclei to halve will always be the same. The number of nuclei that have not yet decayed diminishes very rapidly with the number of half-lives that pass. After ten half-lives, for instance, the radioactivity will have gone down by a factor of 1000.
Nuclei which decay easily have shorter half-lives, while those that have more difficulty last for longer.
At any given moment, the number of remaining nuclei, the number of decays taking place and the number of various types of radiations emitted are all proportional and decrease at the same rate. This mathematical relationship with time allows for a calculation of the half-life. In fact, while it is almost impossible to count the number of nuclei, the decay process can be followed quite easily by measuring the radiations detected with, say, a Geiger counter .
Half-life is a convenient way to assess the rapidity of a decay, but it should not be confused with the average life span of a radioactive nucleus. This average life span, in the case of a simple radioactive decay, is 1,443 times the half-life.
Other articles on the subject « Radioactive decay law »
Radioactive Activity
The decay rate and the rate of radiation emitted The activity of a sample of radioactive matter i[...]
Radioactive Series
A long Radioactive Lineage A certain number of natural radioactive nuclei are still present on Ea[...]
Radioactive Equilibrium
An equilibrium as old as the Earth Radioactive lineage is the term given to the chain of successi[...]